The front window is 195.58 cm wide by 131.45 cm tall, and is reasonably centered at x=2.33cm=x(target) and y=0.The points C2, C4, C5, and C6, given in the table below, define the location and orientation of the front window plane only, although these four points are actually outside of the window perimeter. Therefore these points should not be used to calculate the extent or dimensions of the window.
| X | Y | Z | |
| Point | (cm) | (cm) | (cm) |
| C2 | 2.6365 | -68.6753 | 502.1198 |
| C4 | 103.0741 | -2.8505 | 501.7966 |
| C5 | -97.7327 | -3.4720 | 501.3121 |
| C6 | 2.9695 | 67.9520 | 501.6002 |
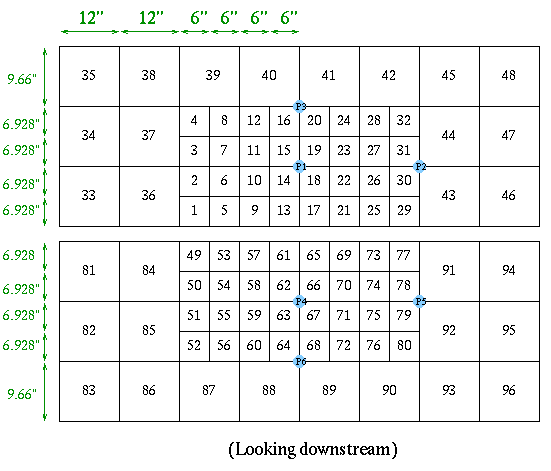
If you were to look at each mirror plane from an infinite distance, you would see the following:

When you look in 3 dimensions however the mirror geometry is a little bit more complicated due to the curvature of the mirrors. The projections of points P1 through P6 onto the effective mirror plane are given in the table below. The absolute position and orientation within the MPS coordinate system is accurate to about 1/16" (0.16 cm) although the relative positions of these points P1-P6 (relative to each other) are accurate to perhaps a few mils.
| X | Y-effective | Z-effective | |
| Point | (cm) | (cm) | (cm) |
| P1 | 2.332 | 31.343 | 568.500 |
| P2 | -58.608 | 31.335 | 568.445 |
| P3 | 2.319 | 61.884 | 585.833 |
| P4 | 2.249 | -30.002 | 567.519 |
| P5 | -58.730 | -29.858 | 567.429 |
| P6 | 2.256 | -60.508 | 585.006 |
The following figure attempts to illustrate the effective mirror plane:
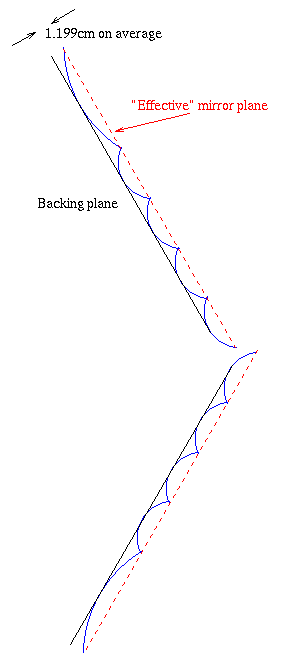
However the 3-dimensional geometry is not so simple, because different mirrors curve different amounts depending on the focus, and because the mirror corners are displaced further from the backing plane than the rest of the edges. The effective mirror plane is calculated using an average displacement of the mirror edge from the mirror backing plane, which could be off by +/- 1/8" or so from the true mirror edge.